§ 2.6. From homotopy to deck transformations for the cubic spherical manifolds.

We now describe the glue algorithm due to Everitt [11] for the cubic spherical manifold $N2$ . It prescribes both the face gluings and the edge gluing according to the following schemes.

¶ Face gluings.

After correction of an error in [39] eq. 9,

$F3\cup F1,\; F4\cup F2,\; F6\cup F5.$

(16)

¶ Edge gluing scheme.

Directed edges in a single line are glued.

$\left[\begin{array}[]{lll}1&3&4\\ 2&6&\overline{9}\\ 5&7&\overline{10}\\ 8&11&\overline{12}\\ \end{array}\right]$

(17)

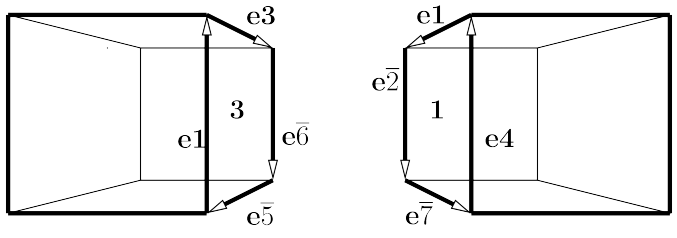
Consider now the first homotopic glue generator $F3\cup F1$ . Following the gluing schemes, we get the correspondence shown in Fig. 23. By use of Fig.18, each face with its edges determines the position and orientation of a copy of the cubic prototile. When the two copies touch one another, they determine a unique relative position and orientation. The isomorphic deck generator is the rotation of the 3-sphere which yields the transformation between these two positions. On the left in Fig. 22 we represent this deck generator by the positions of the prototile and its right-hand neighbour.

Figure 23. First deck generator of the cubic manifold N2: Left and right faces $3$ and $1$ bounded by directed edges $es$ according to Fig 18. The two faces and sets of edges appear as prescribed by the Everitt glue algorithm. Moreover they determine uniquely the positions of two copies of the cube. The relative position of these copies when they touch one another and share their faces determines the isomorphic deck generator, compare the right-hand neighbour for $N2$ on the left of Fig. 22.